Approach
Selected Publications
Nonlinear phononic control and emergent magnetism in Mott insulating titanates
Optical control of structure-driven magnetic order offers a platform for magneto-optical terahertz devices. We control the magnetic phases of d1 Mott insulating titanates using nonlinear phononics to transiently perturb the atomic structure based on density functional theory (DFT) simulations and solutions to a lattice Hamiltonian including nonlinear multimode interactions. We show that magnetism is tuned by indirect excitation of a Raman-active phonon mode, which affects the amplitude of the TiO6 octahedral rotations that couple to static Ti-O Jahn-Teller distortions, through driven infrared-active modes of LaTiO3 and YTiO3. The mode excitation reduces the rotational angle, driving a magnetic phase transition from a ferromagnetic (FM) to G-type antiferromagnetic (AFM) state. A novel A-type AFM state hidden in the bulk equilibrium phase diagram emerges as a dynamically accessible optically induced phase under multimode excitations. Our work shows that nonlinear phononics can stabilize phases inaccessible to static chemical substitutions or lattice strains.
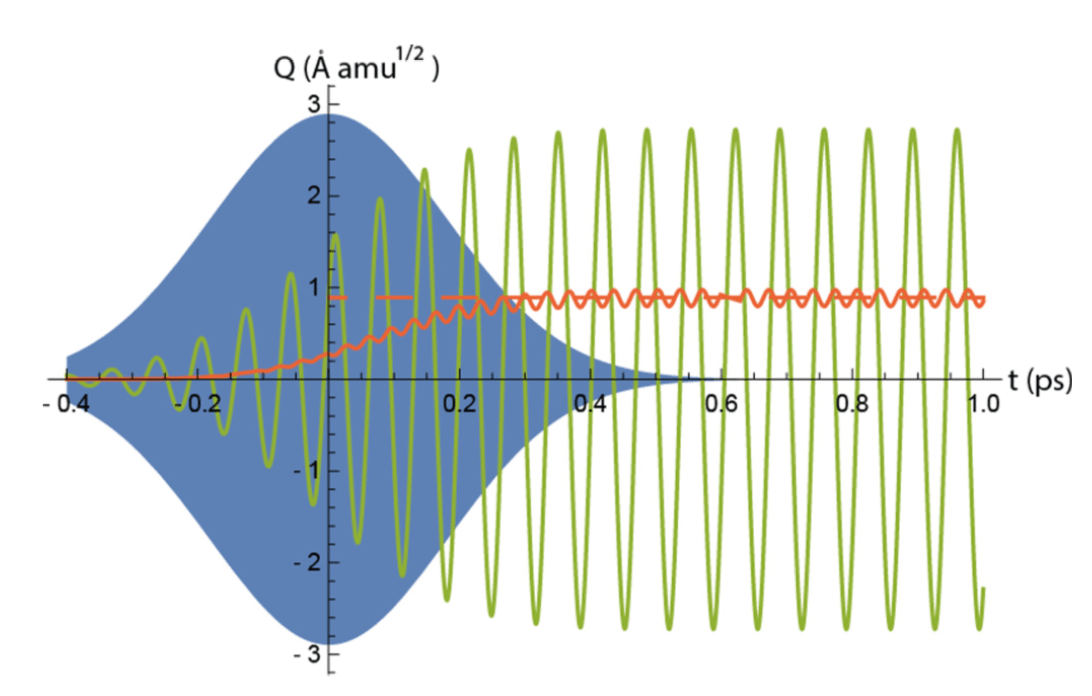
Figure: Time evolution of the Ag (25) mode (red) and the B1u (48) mode (green). The laser pulse envelope is schematically shown as the blue filled curve. The dashed line denotes the displacive vibrational center of the Ag (25) mode after pumped by a laser pulse with ω = 14.5 THz and I = 18 MV/cm (damping is neglected).
Role of orbital filling on nonlinear ionic Raman scattering in perovskite titanates
The linear and nonlinear phononic interactions between an optically excited infrared (IR) or hyper-Raman mode and a driven Raman mode are computed for the d0 (CaTiO3) and d1 (LaTiO3) titanates within a first-principles density functional framework. We calculate the potential energy surface expanded in terms of the Ag or B1g mode amplitudes coupled to the Au or the B3u mode and determine the coupling coefficients for these multimode interactions. We find that the linear-quadratic coupling dominates the anharmonicities over the quadratic-quadratic interaction in the perovskite titanates. The IR and Raman modes both modify the electronic structure with the former being more significant but occurring on a different time scale; furthermore, the coupled-mode interactions lead to sizable perturbations to the valence bandwidth (∼100 meV) and band gap (∼50 meV). By comparing the coupling coefficients of undoped CaTiO3 and LaTiO3 to those for electron-doped (CaTiO3) and hole-doped (LaTiO3) titanates, we isolate the role of orbital filling in the nonlinear coupling process. We find that with increasing occupancy of the d manifold, the linear-quadratic interaction decreases by approximately 30% with minor changes induced by the cation chemistry (that mainly affect the phonon mode frequencies) or by electron correlation. We identify the importance of the Ti-O bond stiffness, which depends on the orbital filling, in governing the lattice anharmonicitiy. This microscopic understanding can be used to increase the nonlinear coupling coefficient to facilitate more facile access of nonequilibrium structures and properties through ionic Raman scattering processes.

Figure: Energy profiles for the nonlinear coupling between two phonon modes. (a)–(d) Energy profiles for the two Ag modes under the excitation of the IR B3u mode in CTO and LTO. (e)–(h) Energy profiles for the B1g mode and the two Ag modes under the excitation of the hyper-Raman Au mode. Note that in the top panels all curves are overlapping, indicating that the B1g mode is decoupled from the Au mode by symmetry. Note that here we show the Ag (1) mode for CTO and the Ag (2) mode for LTO, because the corresponding couplings are stronger. The inset in the left panel shows the predicted Q∗R dependence on the IR mode amplitude from Eq. (2) (curves) and the DFT computed data (symbols).